
Alongside that, it is also used to determine the lattice spacings of crystals.Bragg’s Law is useful for conducting the measurements of wavelengths.There are various applications of Braggs Law of diffraction such as – Substituting equation 2 in equation 1, we get, nλ = 2d sinθ Now, considering d as the hypotenuse of the right-angled triangle, ABz, it is clear that AB is the opposite of the angle θ. Here is the derivation of Bragg’s equation, The extra distance it travelled is referred to as the integral multiple of the wavelength.AB + BC can be considered the distance travelled by the second beam.The second beam scatters when it reaches point B.When they eventually get to point z, they travel upwards after striking the surface.The incident beams are parallel to each other before they reach point, z.To derive Bragg's equation, let us consider a figure to observe the phases of beams that coincide when the incident angle is equivalent to the angle of reflection. which represents the order of reflection.īraggs law Statement and Derivation – Bragg's Law states that when X-rays or other electromagnetic radiation waves interact with a crystal lattice, the waves undergo constructive interference if the following condition is met: the path difference between waves reflected from adjacent crystal lattice planes is equal to an integer multiple of the wavelength of the incident radiation. Bragg worked out and gave a mathematical relation to determining the inter-atomic distance from the X-ray diffraction pattern. If n = 2 and λ > d, then there is no solution because the sine of an angle can't be more than 1.Įxample 2: In Bragg's equation for diffraction of X-rays, n represents? (2 Marks) Reason: Bragg's equation is given by nλ=2dsinθ. The diffraction analysis is the resulting wave interference, and this analysis is known as Bragg diffraction.Įxample 1: Justify the Following: Braggs equation has no solution if n = 2 and λ > d.The analysis of diffraction and the interference of the resulting wave is referred to as Bragg diffraction.The fields of wave that are re-emitted interfere with each other constructively and destructively, creating a pattern of diffraction on a detector or a film.The same process occurs when neutron waves are scattered via nuclei or a coherent spin interaction along with an isolated electron.When the path difference equals a whole number, n, of wavelength, λ, constructive interference will occur. When the X-rays are incident on a crystal surface, the angle of incidence (θ), will reflect back with the same angle of scattering, θ. The video below explains this: Bragg’s Law Detailed Video Explanation:īragg's equation for the diffraction of an X-ray is written as follows – Bragg’s Law helps to determine the angles formed by coherent and incoherent scattering from a crystal lattice.Bragg’s law basically explains the relation between an X-ray light shooting and its reflection from a crystal surface.This phenomenon is called Rayleigh scattering.The movement of the charges releases waves of the same frequency as X-rays but are slightly blurred due to various effects.When X-rays are incident on an atom, they form an electronic cloud moving like an electromagnetic wave.θ is the angle of incidence and scattering.īragg's law in Physics states that when an X-ray is an incident on a crystal surface, its angle of incidence, θ reflects back with the same angle of scattering θ.d is the distance between the atomic layers in the crystal lattice.λ is the wavelength of the incident X-ray beam.
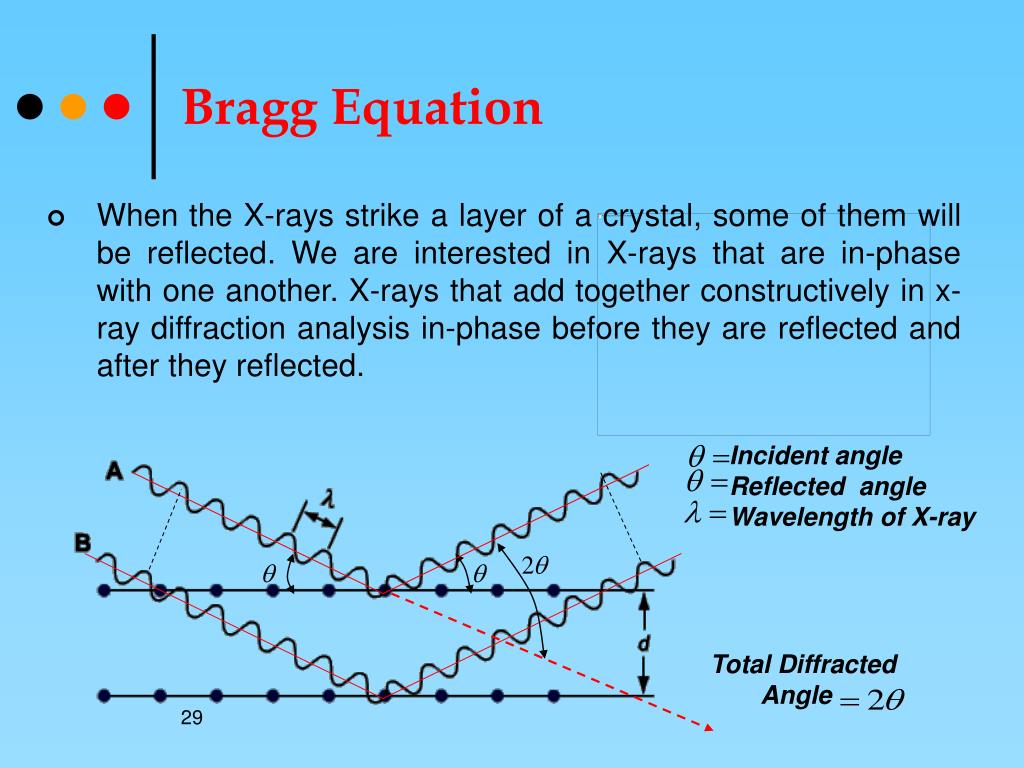
n is an integer representing the order of reflection.Constructive interference will occur when path difference ‘d’ is equal to the whole number ‘n’ of the wavelength.” Mathematically, Bragg’s law equation can be represented as, The derivation of Bragg's Law involves the phases of beams that coincide when the incident angle is equal to the angle of reflection.īragg’s law states that “ When a beam of x-ray is incident on a crystal at an angle θ, the reflected beam of x-ray also has the same angle of scattering θ.When the X-rays are incident on an atom, it moves the electronic cloud just like an electromagnetic wavedoes.Bragg’s Law is a special case of Laue diffraction to understand the angles of coherent and incoherent scattering from a crystal lattice.The law is based on the principles of constructive interference and diffraction. \nonumber \] If we use nonrelativistic momentum, we obtain the same result because 1 eV is much smaller than the rest mass of the electron.Bragg's Law describes the relationship between the angles of incidence and reflection of electromagnetic waves as they interact with a crystal lattice structure.


 0 kommentar(er)
0 kommentar(er)
